Why EVERY Sports Scientist Uses Z Scores!

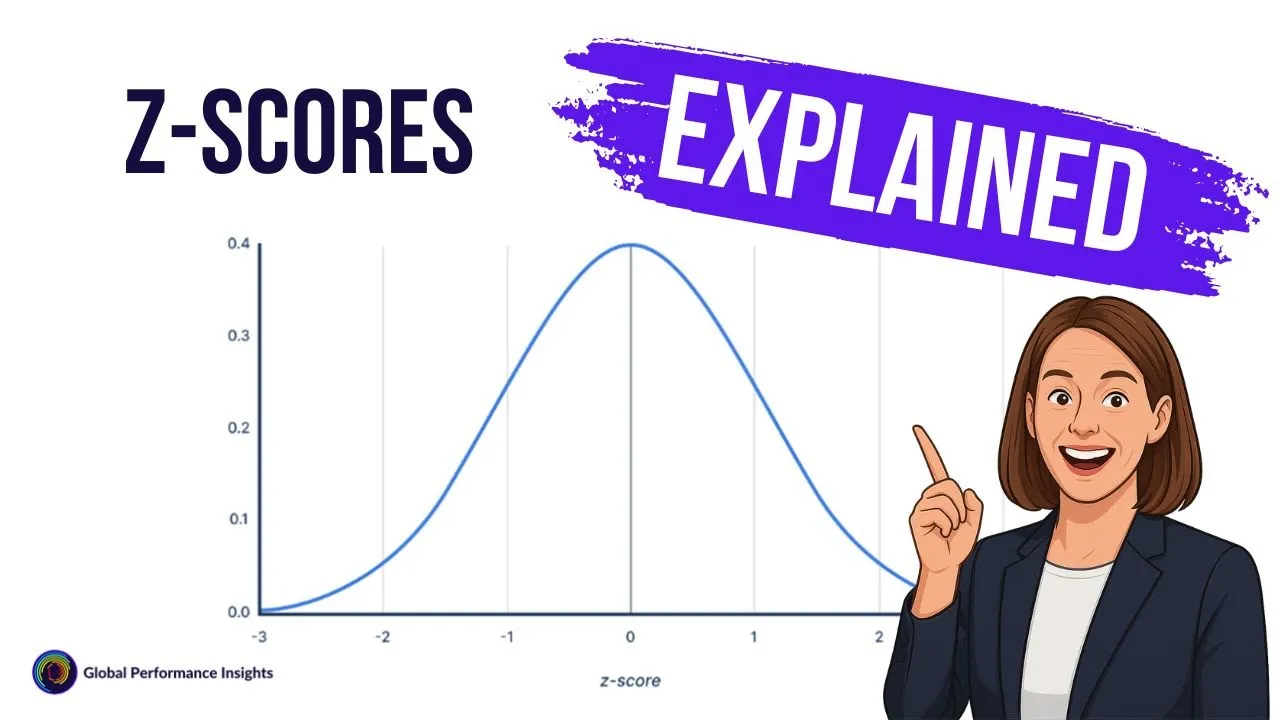
A Z-score is one of the most useful. statistics a sports scientist can use. We are always wanting to know. how does a number compare to a previous set of numbers. That might be comparing an. individual athlete wellness score for today compared to a period. of time for themselves previous. It might be comparing an athlete, in terms of their physical testing results to the rest of the group. Now sometimes we might use a percentage calculation to flag whether a amount was 10 or 20% higher or lower than normal, but we're often dealing with numbers with different scaling properties. And we're also dealing with athletes. who some athletes might score or report a real varied range of numbers. And other types of tests or athletes might be a lot more narrow. So we want to somehow take into account the norms for their data. And the Z- score, the Z score in, American, or a standardised score as it's also known, allows us to do that. The number it gives us is actually the number of standard deviations away from the mean. So here we have a standard normal, distribution curve also known as a bell curve, which tells us that more trials. and results occur around the mean and less of those results occur at the. outer edges, the tails of that data. And this is what a Z- score is based, upon because plus one to minus one of the Z- score, the plus showing that the. score that we're comparing is higher than the average in the dataset we're comparing it to and negative is reverse. So that means it's lower than. Plus 1 to minus 1 z-scores wi ll represent. about 68% of the values in your dataset. Plus two to minus two. will represent about 95%. And this is linked to that p number of being 0.5 for statistical significance. And technically 99.7% of the values are expected to be within this plus three to minus three Z- score range for a normally distributed dataset. Now if this formula looks scary Don't. don't worry. It really isn't. And you'll be able in a few moments. After watching this video, you'll know this formula inside out. So, this is what all those, fancy symbols represent. We take the score that we want to calculate the z-score. That's our x and we take away. the mean of our comparison group. And then we divide it by our standard deviation of our comparison group. And that then gives us our Z -score. So let's start with a very simple example. Let's say that we have a score for an, athlete today of 9.4, whatever that might be, it might be a test, a speed,, a wellness score, but today is 9.4. Their average is 8.1. And their standard deviation for that. range that we are comparing it to is 1.2. So to calculate our Z score, we want, to do today for the individual subject, subtracted by their average, and then, divide that by the standard deviation. And that gives us a Z- score of 1.1. So we know that 9.4 is higher than their average of 8.1. And it's 1.1 standard deviations for their data set away from their average. So remember the Plus one to minus one is about 68% of the values. So this is, it's higher than. average, but we wouldn't say this is Statistically higher than average. Often people have their flags set plus or minus 1.5. or plus or minus two, perhaps you might, have some sort of amber flag that is set at Plus or minus one z- score, And, in which case, this example would flag. Now I'll be honest Excel actually has a standardized function specifically for Z- scores. And we can see that that formula. is equals standardized X mean and standard deviation, the three. components that we would expect. But for the sake of this video and, keeping reinforcing that calculation, I'm going to not use a standardized

formula and keep almost calculating it manually so we can keep reinforcing that. Now we'll move on to looking at how, does one athlete compare to a group? So here I have a number of different, test results for 10 different athletes. First thing I'm gonna do is just calculate. the average and standard deviations using the Excel functions for each metric. So we have those to refer to. Now I'm gonna mirror this table here. And create a replica, but this. time using the Z- scores for each corresponding result. So we'll start with mass of a, and if you remember, our formula is their score, take away the group average,, divided by the standard deviation. So we see a result a Z- score of 1.37. Their mass is 130 compared to the group average of 1 0 3 0.5. So they are 1.37. Z- Scores higher than the group. And if you remember from the bell. curve distribution plus one to minus one is about 68% within a, normally distributed data set. So this is above average. It wouldn't be statistically significant. Now, if I was gonna drag this. down, all the cells in the formula are gonna shift with it. So we are gonna get an error because, now it's not looking in the right place for the average and standard deviation. So a fantastic little trick in, Excel, is to use the dollar sign within a formula to anchor the cells. So if we wanted to anchor a, column we would put a dollar sign before the letter, the column. If we want to anchor a row, We put a dollar sign before the number. In this case, we do, want to anchor the rows. We want it to always be looking at, rows 13 and 14 for the average and the standard deviation, respectively. So in our top formula here, I'm. gonna leave B2 alone because we want that to shift as we move this formula and copy and paste it. But before the. 13, I'm gonna put a dollar sign. And before the 14, I'm gonna put a dollar sign. And now when we drag our formula down or copy and paste it, however you wish to do it. We see the errors gone. If I select this one, we are now, getting Athlete F's score compared to the average and the standard deviation every time in every row. So I can also now drag this across. And we see we get the Z- score for every. metric that we've got here in the table. For instance here. We've got for athlete A, their strength in column H. Being compared to the group, average and standard deviation. Now, perhaps what I wanna do is, add some conditional formatting to this table so that my flags. are automatically highlighted. So I'll highlight this array and I'll go, into conditional formatting and new rule. Lots of different ways that we can format. based on different types of conditions. In this case, I'm going to do only. format cells with a cell value greater than or equal to 1.5. So if someone's test score is 1.5 z. score then I want that highlighted and I'm just gonna pick for now orange. And we can see that our peak power for athlete B. Is higher than 1.5. Z- scores. And also a number of our results for athlete F are also highlighted. Thank you to action apps for sponsoring this video. Action apps have revolutionized. asset management systems with their unparalleled flexibility and. superior technology trusted by top sports organizations worldwide. Their cutting edge data visualization, and analytics reports, along with customized apps, empowers you to. make better, more informed decisions. Check out action apps.co uk for more information. Now I wanna show a second way that we can calculate it so I'm just gonna hide this for a moment and, we're gonna do the same thing. Imagine we don't have our average and standard deviation on the spreadsheet. What we can do is we can nest that. calculation within the formula itself. So we start with the score in question. We wanna take it away remember from the group average. And in this case, I'm gonna nest that

average calculation within this formula. And then we're gonna divide it. Same thing. Including the standard. deviation in the formula. And we can see that we get our 1.37 Z-score for athlete A's mass again. But if I now copy and paste, this formula. Drag it down. Although we're getting numbers. these technically are incorrect because the average and standard. deviation group is moving down. Every time we copy again. So we wanna use our dollar. sign trick again, to anchor the formula. So we want it to be B2 to B 11 in relation to our average and standard deviation calculation. We want to anchor the row. Not the column. So we're putting it before. The number. In this case now when we drag it down, We can see that we've got z-score,. and again, our conditional formatting is showing up and looks. very similar if I unhide our first group and we can directly compare. That as we should have, we've got the. exact same z- scores being calculated and the same ones being flagged. So it just depends on the. structure of your spreadsheet. Something that I like to do personally, rather than have our average and our standard deviation below. You can put it above, almost as a column. header, particularly if you know, you're gonna keep adding to the data set below. But one example where that might not be relevant. Here, we're gonna look. at one athlete over time. Now this could be an R p E score. It could be a wellness score. I want to compare today to a set period of time previously. So in this instance, I. don't wanna know today. compared to the entire season. I want to have more of a rolling, average, so maybe I wanna compare to today to the last two weeks. So the last 14 days. I actually want to compare today here to the average of the previous 14 days. And divide that by the standard, deviation of that same timeframe. And we can see now. We get our Z three is 0.7 standard, deviations lower because it's negative. And now when I drag this down, You can see that our Point of comparison also moves with it in a in a way that is correct. Unlike that previous. example we were looking at. And again, if I add some conditional formatting here, greater than 1.5, we can see a number of flags here. Where the athlete now is, logging four and five. Whereas before again, I could put the. conditional formatting for minus, and we could see here in this timeframe. that for them compared to themselves, over a select period of time, they did go through a period of time where they were reporting a lower number. And now, reporting generally much higher number. Scores are way more valuable than just the score and some conditional formatting. They also can help us with some of. our visualization and give us some really meaningful charts without, people and the reader having to understand the underlying statistics. Let's take a look at a couple of examples of data visualizations from today's sponsor Action Apps. So here we just have some demo data in a demonstration account with action apps. And if we think back to the testing. data we were looking at ourselves earlier, this really does suit. visualization in a radar plot whereby we can compare an individual athlete's. results across a number of different tests, either to the whole squad, we. can compare them to a position average or we can plot them against another, athlete as shown here, for example. We might wanna plot our Z- scores, over time, particularly those that we are collecting on a regular basis, such as with our subjective data like RPE or wellness. So we can see here a trend over a period of time, but we can also, in this instance, click in and explore. the individual Z-score trends for different athletes over the timeframe,. in this case here to their own data set..